Bei Wang

PDF
DBI: ABI Innovation: A Scalable Framework for Visual Exploration and Hypotheses Extraction of Phenomics Data using Topological Analytics

Award Number and Duration

NSF DBI 1661375

August 1, 2017 to July 31, 2020 plus 1-year NCE

This project webpage highlights the results of the project NSF DBI 1661375 yielded by (partial or complete) efforts at the University of Utah.

For collaborative project NSF DBI 1661348, and collaborative project directions, please see Collaborative Project Website: http://tdaphenomics.eecs.wsu.edu/.

Point of Contact and PI

Bei Wang (PI, University of Utah)
Assistant Professor
School of Computing and Scientific Computing and Imaging Institute
University of Utah
beiwang AT sci.utah.edu
http://www.sci.utah.edu/~beiwang

Collaborators

Anantharaman Kalyanaraman (PI, Washington State University)
Bala Krishnamoorthy (Co-PI, Washington State University)
Zhiwu Zhang (Co-PI, Washington State University)
Patrick S. Schnable (PI, Iowa State University)
Larry Holder (Washington State University)

Overview

Understanding how gene by environment interactions result in specific phenotypes is a core goal of modern biology and has real-world impacts on such things as crop management. Developing and managing successful crop practices is a goal that is fundamentally tied to our national food security. By applying novel computational visual analytical methods, this project seeks to identify and unravel the complex web of interactions linking genotypes, environments and phenotypes. These methods will first need to be designed and developed into usable software applications that can handle large volumes of crop phenomics data. High-throughput sensing technologies collect large volumes of field data for many plant traits, such as flowering time, related to crop development and production. The maize cultivars used here come from multiple genotypes that have been grown under a variety of environmental conditions, in order to give the widest range of conditions for understanding the interactions. The resulting data sets are growing quickly, both in size and complexity, but the analytical tools needed to extract knowledge and catalyze scientific discoveries have significantly lagged behind. The methodologies to be developed in this project represent a systematic attempt at bridging this rapidly widening divide. The project is inherently interdisciplinary, involving close research partnerships among computer scientists, plant scientists, and mathematicians. The research outcomes will be tightly integrated with education using a multipronged approach that includes, among others, postdoctoral and student training (graduates and undergraduates), curriculum development for a new campus-wide interdisciplinary undergraduate degree in Data Analytics, conference tutorials for training phenomics data practitioners, and contribution to the recruitment and retention of underrepresented minorities (particularly women) in STEM fields through the Pacific Northwest Louis Stokes Alliance for Minority Participation.

This project will lead to the design and development of a new, scalable, visual analytics platform suitable for hypothesis extraction and refinement from complex phenomics data sets. Focus on hypothesis extraction is critical in the context of phenomics data sets because much of the high-throughput sensing data being generated in crop fields are generated in the absence of specifically formulated hypotheses. Extracting plausible hypotheses from the data represents an important but tedious task. To this end, this project will apply and develop new capabilities using emerging advanced algorithmic principles, particularly from the branch of mathematics called algebraic topology that studies shapes and structure of complex data. The research objectives are three-fold. First, the project will employ and extend emerging algorithmic techniques from algebraic topology to decode the structure of large, complex phenomics data. Second, an interactive visual analytic platform will be developed to facilitate knowledge discovery using the extracted topological structures. Lastly, the quality and validity of a new visual analytic platform designed by this team will be tested using real-world maize data sets as well as simulated inputs as testbeds. The developed framework will encode functions for scientists to delineate hypotheses of three kinds: i) genetic characterization of single complex traits; ii) genetic characterization of multiple traits that share potentially pleiotropic effects; and iii) decoding and detailed characterization of genotype-by-environmental interactions, in particular, through a collaborative pilot study of maize flowering and growth traits. The expected significance of the proposed work is that biologists will be able to extract different types of testable hypotheses from plant phenomics data sets by employing a new class of visual analytic tools, and thus obtain a deeper understanding of the interactions among genotypes, environments and phenotypes. The project is potentially transformative in two ways: i) it will introduce advanced mathematical and computational principles into mainstream phenomic data analysis; and ii) it will usher in a new era where biologists spearhead data-driven hypothesis extraction and discovery with the aid of interactive, informative, and intuitive tools. The project will have a direct impact on the state of software in phenomics for fundamental data-driven discovery. To facilitate broader community adoption, the project will integrate the tools into the CyVerse Institute, and to a community phenomics software outlet. It will also lead to the development of automated scientific workflows. Project website: http://tdaphenomics.eecs.wsu.edu/.

Publications

Year 4 (2020 - 2021)
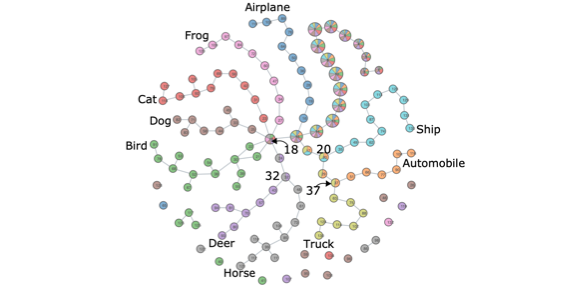
	Pheno-Mapper: An Interactive Toolbox for the Visual Exploration of Phenomics Data. Youjia Zhou, Methun Kamruzzaman, Patrick Schnable, Bala Krishnamoorthy, Ananth Kalyanaraman, Bei Wang. Proceedings of the 12th ACM Conference on Bioinformatics, Computational Biology, and Health Informatics (ACM BCB), Article No. 20, pages 1-10, 2021. DOI: 10.1145/3459930.3469511
	Mapper Interactive: A Scalable, Extendable, and Interactive Toolbox for the Visual Exploration of High-Dimensional Data. Youjia Zhou, Nithin Chalapathi, Archit Rathore, Yaodong Zhao, Bei Wang. IEEE Pacific Visualization Symposium, 2021. DOI: 10.1109/PacificVis52677.2021.00021 arXiv:2011.03209.
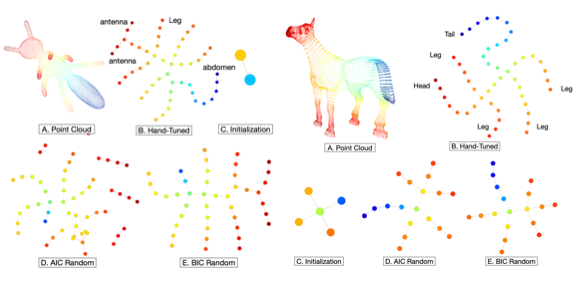
	Adaptive Covers for Mapper Graphs Using Information Criteria. Nithin Chalapathi, Youjia Zhou, Bei Wang. Workshop on Applications of Topological Data Analysis to Big Data at the IEEE International Conference on Big Data, 2021.
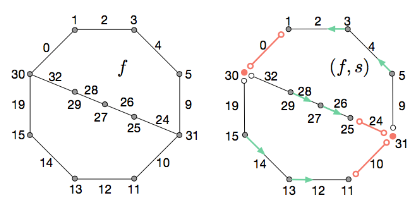
	Discrete Stratified Morse Theory: Algorithms and A User's Guide Kevin Knudson and Bei Wang. Discrete & Computational Geometry, accepted, 2021.
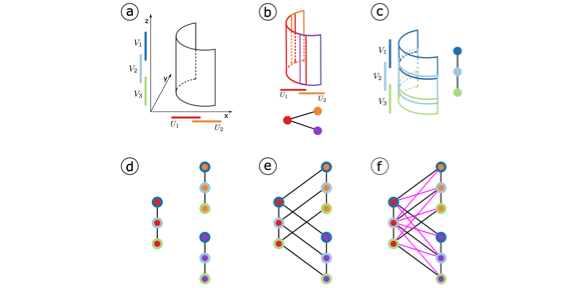
	Stitch Fix for Mapper and Topological Gains. Youjia Zhou, Nathaniel Saul, Ilkin Safarli, Bala Krishnamoorthy, Bei Wang. Research in Computational Topology 2, accepted, 2021.
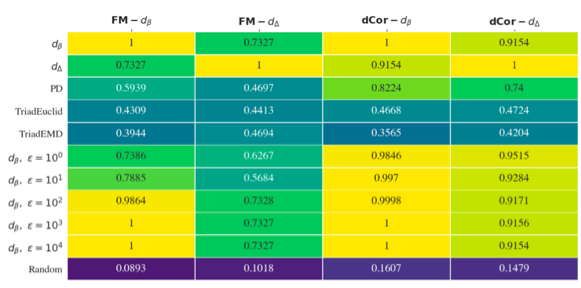
	Graph Pseudometrics from a Topological Point of View. Ana Lucia Garcia-Pulido, Kathryn Hess, Jane Tan, Katharine Turner, Bei Wang, Naya Yerolemou. Research in Computational Topology 2, accepted, 2021.
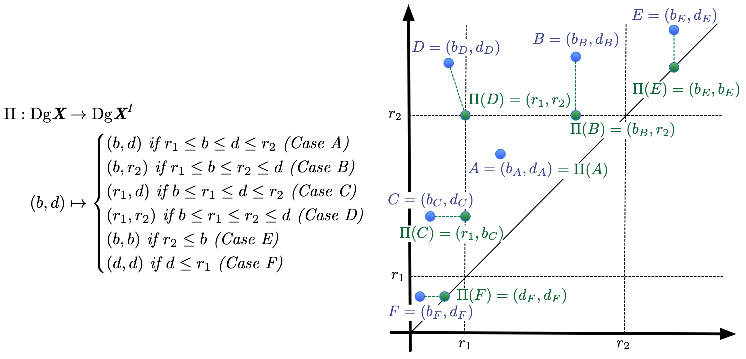
	Local Versus Global Distances for Zigzag Persistence Modules. Ellen Gasparovic, Maria Gommel, Emilie Purvine, Radmila Sazdanovic, Bei Wang, Yusu Wang, Lori Ziegelmeier. Research in Computational Topology 2, accepted, 2021. arXiv:1903.08298.
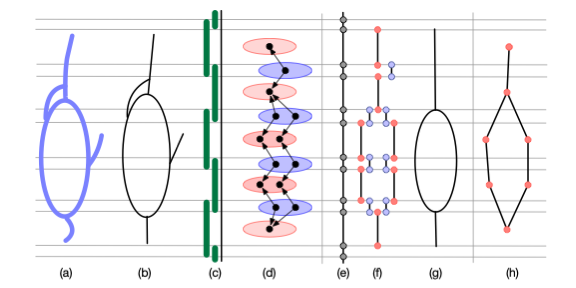
	Probabilistic Convergence and Stability of Random Mapper Graphs. Adam Brown, Omer Bobrowski, Elizabeth Munch, Bei Wang. Journal of Applied and Computational Topology, 5, pages 99-140, 2021. DOI:10.1007/s41468-020-00063-x arXiv:1909.03488.
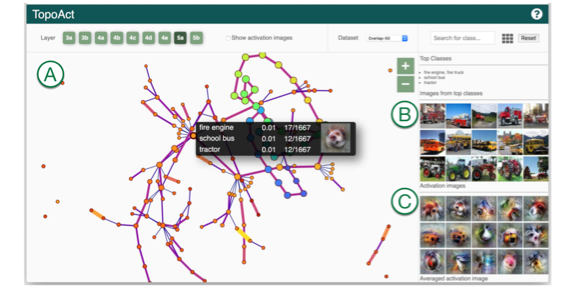
	TopoAct: Visually Exploring the Shape of Activations in Deep Learning. Archit Rathore, Nithin Chalapathi, Sourabh Palande, Bei Wang. Computer Graphics Forum, 40(1), pages 382-397, 2021. Supplemental Material. DOI: 10.1111/cgf.14195 arXiv:1912.06332.
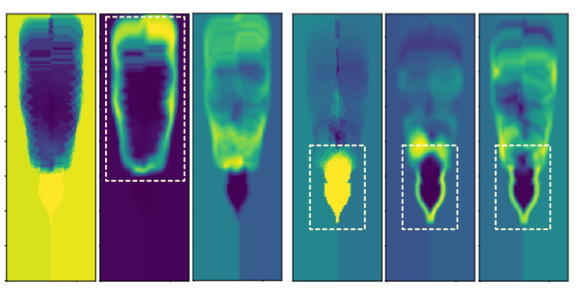
	Uncertainty Visualization of 2D Morse Complex Ensembles Using Statistical Summary Maps. Tushar Athawale, Dan Maljovec, Lin Yan, Chris R. Johnson, Valerio Pascucci, Bei Wang. IEEE Transactions on Visualization and Computer Graphics, 2020. DOI: 10.1109/TVCG.2020.3022359
Year 3 (2019 - 2020)
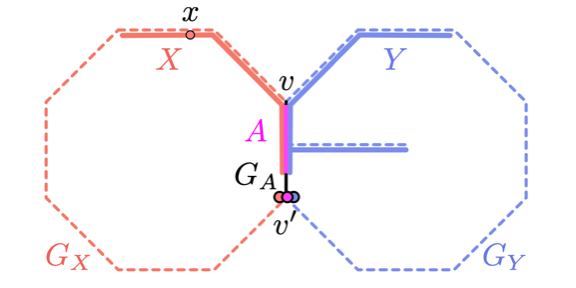
	On Homotopy Types of Vietoris--Rips Complexes of Metric Gluings. Michal Adamaszek, Henry Adams, Ellen Gasparovic, Maria Gommel, Emilie Purvine, Radmila Sazdanovic, Bei Wang, Yusu Wang and Lori Ziegelmeier. Journal of Applied and Computational Topology, 4, pages 425-454, 2020. DOI:10.1007/s41468-020-00054-y arXiv:1712.06224.
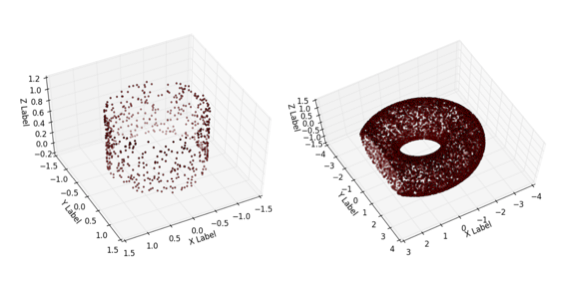
	Topological Inference of Manifolds with Boundary. Yuan Wang, Bei Wang. Computational Geometry: Theory and Applications, 88(101606), 2020. DOI:10.1016/j.comgeo.2019.101606 arXiv:1810.05759
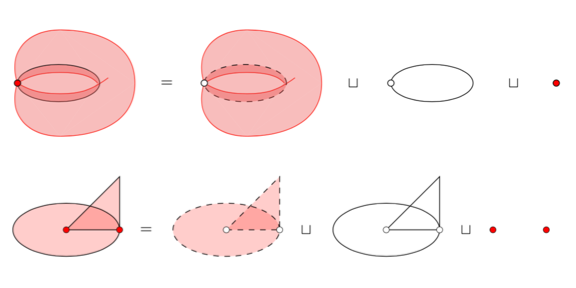
	Sheaf-Theoretic Stratification Learning From Geometric and Topological Perspectives. Adam Brown and Bei Wang. Discrete & Computational Geometry, 2020. DOI:10.1007/s00454-020-00206-y arXiv:1712.07734
	State of the Art in Time-Dependent Flow Topology: Interpreting Physical Meaningfulness Through Mathematical Properties. Roxana Bujack, Lin Yan, Ingrid Hotz, Christoph Garth, Bei Wang. Eurographics Conference on Visualization (EuroVis) STAR Computer Graphics Forum, 2020. DOI: 10.1111/cgf.14037
	The Relationship Between the Intrinsic Cech and Persistence Distortion Distances for Metric Graphs. Ellen Gasparovic, Maria Gommel, Emilie Purvine, Radmila Sazdanovic, Bei Wang, Yusu Wang, Lori Ziegelmeier. Journal of Computational Geometry, 10(1), pages 477-499, 2019. DOI: 10.20382/jocg.v10i1a16 arXiv:1812.05282

Year 2 (2018 - 2019)
	A Kernel for Multi-Parameter Persistent Homology. René Corbet, Ulderico Fugacci, Michael Kerber, Claudia Landi, Bei Wang. Shape Modeling International (SMI), 2019. Computers & Graphics: X, 2019. arXiv:1809.10231. Best Paper Award at SMI 2019!
	Persistent Homology Guided Force-Directed Graph Layouts. Ashley Suh, Mustafa Hajij, Bei Wang, Carlos Scheidegger, Paul Rosen IEEE Transactions on Visualization and Computer Graphics (TVCG, Proceedings of InfoVis), 26(1), pages 697-707, 2020. DOI: 10.1109/TVCG.2019.2934802 arXiv:1712.05548 Long Video. Short Video.
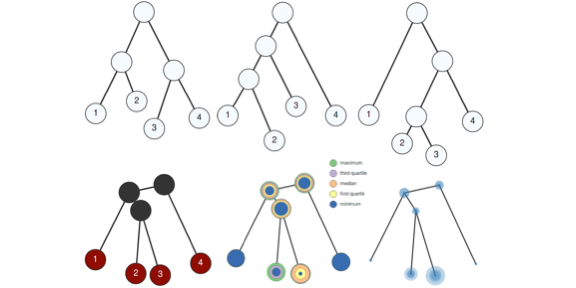
	A Structural Average of Labeled Merge Trees for Uncertainty Visualization. Lin Yan, Yusu Wang, Elizabeth Munch, Ellen Gasparovic, Bei Wang. IEEE Transactions on Visualization and Computer Graphics (TVCG, Proceedings of SciVis), 26(1), pages 832-842, 2020. Supplemental Material. Doi: 10.1109/TVCG.2019.2934242 arXiv:1908.00113 Long Video. Short Video.
Year 1 (2017 - 2018)
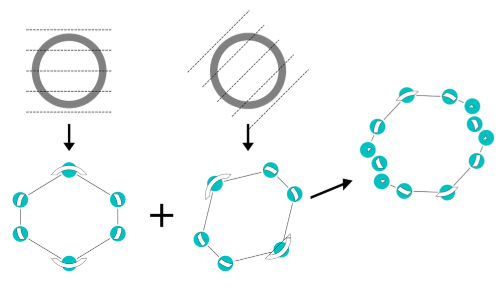
	Stitch Fix for Mapper (Abstract). Bala Krishnamoorthy, Nathaniel Saul and Bei Wang. Computational Geometry: Young Researchers Forum at International Symposium on Computational Geometry (SOCG), 2018. YRF Book of Abstracts
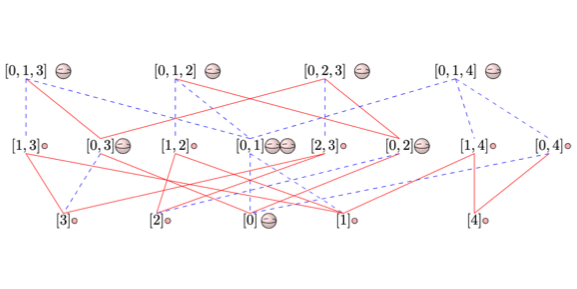
	Discrete Stratified Morse Theory: A User's Guide. Kevin Knudson and Bei Wang. International Symposium on Computational Geometry (SOCG), 2018. DOI: 10.4230/LIPIcs.SoCG.2018.54 arXiv:1801.03183
	Sheaf-Theoretic Stratification Learning. Adam Brown and Bei Wang. International Symposium on Computational Geometry (SOCG), 2018. DOI: 10.4230/LIPIcs.SoCG.2018.14 arXiv:1712.07734
	Vietoris-Rips and Čech Complexes of Metric Gluings. Michal Adamaszek, Henry Adams, Ellen Gasparovic, Maria Gommel, Emilie Purvine, Radmila Sazdanovic, Bei Wang, Yusu Wang and Lori Ziegelmeier. International Symposium on Computational Geometry (SOCG), 2018. DOI: 10.4230/LIPIcs.SoCG.2018.3 arXiv:1712.06224
Manuscripts
	Gazing Into the Metaboverse: Automated Exploration and Contextualization of Metabolic Data. Jordan A. Berg, Youjia Zhou, T. Cameron Waller, Yeyun Ouyang, Sara M. Nowinski, Tyler Van Ry, Ian George, James E. Cox, Bei Wang, Jared Rutter. Manuscript, 2020. bioRxiv:10.1101/2020.06.25.171850v1.
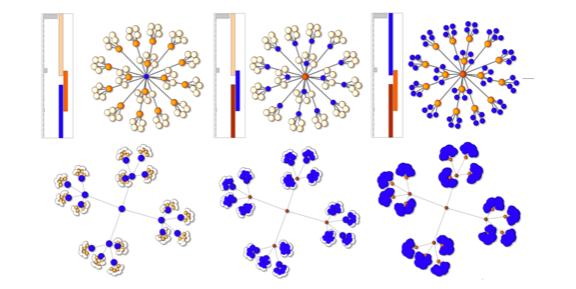
	Mapper on Graphs for Network Visualization. Mustafa Hajij, Bei Wang, Paul Rosen. Manuscript, 2018. arXiv:1804.11242.

Software Downloads

	Mapper Interactive https://mapperinteractive.github.io/ Mapper Interactive provides a library of easily-extendable modules for developing interactive visualization of high-dimensional data using the mapper construction. It is fairly lightweight, and helps to rapidly explore the parameter space of the mapper construction interactively. Mapper Interactive is under active development. Please excuse its appearance (e.g., documentations).
	Pheno-Mapper https://github.com/tdavislab/PhenoMapper Pheno-Mapper is a domain-specific adaptation of Mapper Interactive and specifically targets the analysis and visualization of multi-dimensional phenomics data.
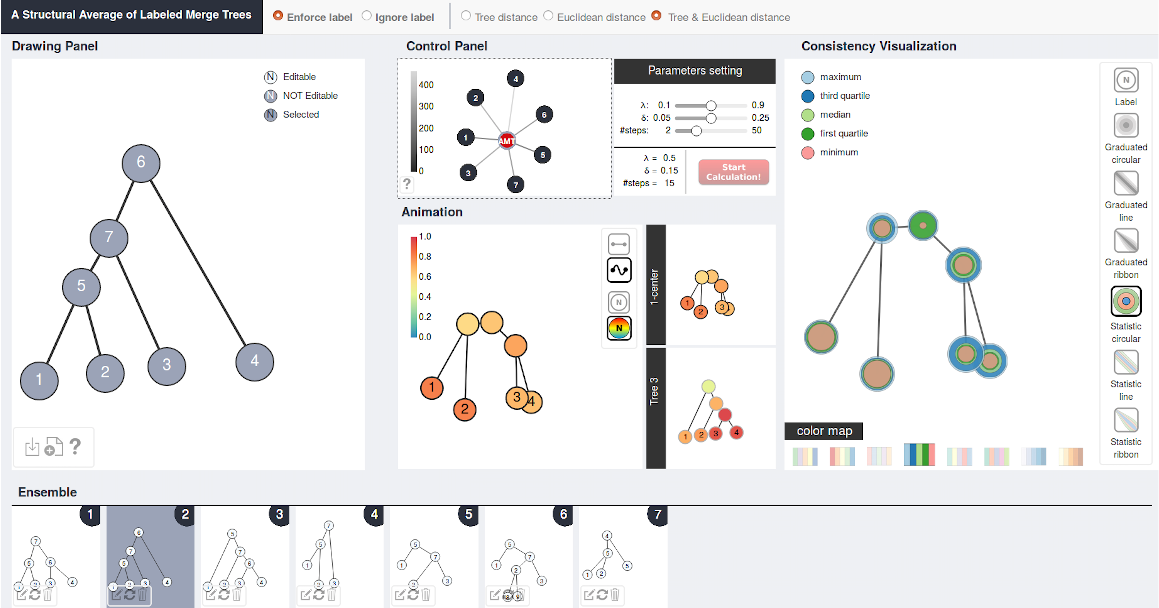
	AMT: Interactive Visualization of Labeled Merge Trees and Their 1-Center https://github.com/tdavislab/amt AMT is an interactive visualization tool that computes an average tree structure for an ensemble of input (merge) trees. It can also be potentially used to compute an average clustering given a collection of hierarchical clustering results. AMT is under active development. Please excuse its appearance (e.g., documentations).
	Metaboverse: Automated Exploration and Contextualization of Metabolic Data. https://github.com/Metaboverse/ Metaboverse is an interactive visualization tool for automated exploration and contextualization of metabolic data. Integrating multi-omic or single-omic metabolic data upon the metabolic network can be challenging for a variety of reasons. Metaboverse seeks to simplify this task for users by providing a simple, user-friendly interface for layering their data on a dynamic representation of the metabolic network. Additionally, it provides several new tools to enable the contextualization of metabolic data. Metaboverse is under active development. Please excuse its appearance (e.g., documentations).
	TopoAct: Visually Exploring the Shape of Activations in Deep Learning. https://github.com/tdavislab/TopoAct/ TopoAct is a visual exploration system used to study topological summaries of activation vectors for deep neural networks. We present visual exploration scenarios using TopoAct that provide valuable insights towards learned representations of image classifiers such as GoogLeNet and ResNet. TopoAct is under active development.

Presentations, Educational Development and Broader Impacts

Year 4 (2020 - 2021)

Nithin Chalapathi Conference Talk (virtual): Adaptive Covers for Mapper Graphs Using Information Criteria at IEEE International Conference on Big Data: Workshop on Applications of Topological Data Analysis to Big Data, December 15, 2021.

Youjia Zhou Conference Talk (virtual): Pheno-Mapper: An Interactive Toolbox for the Visual Exploration of Phenomics Data at ACM-BCB, August 1-4, 2021.

Youjia Zhou Conference Talk (virtual): Mapper Interactive: A Scalable, Extendable, and Interactive Toolbox for the Visual Exploration of High-Dimensional Data at IEEE PacificVis, April 19-22, 2021.

Bei Wang Invited Talk (virtual), Seminar GEOTOP-A: Applications of geometry and topology, August, 2021.

Bei Wang Invited Talk (virtual), ILJU Pohang University of Science Technology (POSTECH) Mathematical Institute for Data Science (MINDS) Workshop on Topological Data Analysis and Machine Learning, South Korea, July, 2021.

Bei Wang Invited Talk (virtual), SIAM Conference on Applications of Dynamical Systems (DS21), Mini-symposium on Topological Signal Processing, May 2021.

Bei Wang Invited Talk (virtual), MSRI (Mathematical Sciences Research Institute) Hot Topics: Topological Insights In Neuroscience, May 2021.

Bei Wang Invited Talk (virtual), Applied Algebraic Topology Research Network (AATRN) Vietoris-Rips Seminar, May 2021.

Bei Wang Invited Talk (virtual), Geometry-Topology Seminar, Oregon State University, May, 2021.

Bei Wang Invited Talk (virtual), Computational Mathematics, Science and Engineering (CMSE) Colloquiums, Michigan State University, Apr. 2021.

Bei Wang Invited Talk (virtual), Meldrum Science Seminar Series, Westminster College, Apr. 2021.

Bei Wang Invited Talk (virtual), CAM Colloquium, Committee on Computational and Applied Mathematics (CCAM), University of Chicago, Mar., 2021.

Bei Wang Invited Talk (virtual), Pacific Northwest National Laboratory (PNNL) Mathematics for Artificial Reasoning in Science (MARS) Seminar Series, Jan. 2021.

Bei Wang Invited Talk (virtual), Joint Mathematics Meetings (JMM) AMS Special Session on Combinatorial Approaches to Topological Structures, Jan. 2021.

Year 3 (2019 - 2020)

Bei Wang Invited Talk (virtual): Topology as a knob for machine learning at MBI Optimal Transport Workshop: Optimal Transport, Topological Data Analysis and Applications to Shape and Machine Learning, July 27-31, 2020.

Lin Yan, Nithin Chalapathi, Bei Wang: Hi-GEAR (Girls Engineering Abilities Realized) Visual Camp, Computer Science -- Data Visualization Board, July 7-9, 2020.

Bei Wang Invited Talk (virtual): TopoAct: Visually exploring the shape of activations in deep learning at GAMES: Graphics And Mixed Environment Seminar, July 2, 2020.

Bei Wang Invited Talk (virtual): TopoAct: Visually exploring the shape of activations in deep learning at Applied Algebraic Topology Research Network, May 20, 2020.

Bei Wang Guest Lecture (virtual) on data visualization for Dr. David Millman's class (graduate and undergraduate students) at Montana State University, April 28, 2020.

Bei Wang Invited Talk: Probabilistic Convergence and Stability of Random Mapper Graphs at Joint Mathematics Meetings AMS Special Session on Applied Topology, Jan. 17, 2020.

Bei Wang Invited Talk: A Structural Average of Merge Trees and Uncertainty Visualization at TDA Lunch, Centre for Topological Data Analysis, University of Oxford, Oct 11, 2019.

Bei Wang Invited Talk: A Structural Average of Labelled Merge Trees at the Institute of Applied Data Science (IADS) at Queen Mary University of London, Oct 9, 2019.

Year 2 (2018 - 2019)

Bei Wang and Bala Krishnamoorthy Workshop Organization: 8th Annual Minisymposium on Computational Topology during the Computational Geometry Week, June 18-21, 2019.

Bei Wang Workshop Organization: Dagstuhl Seminar: Topology, Computation and Data Analysis, May 19 - 24, 2019.

Youjia Zhou Poster Presentation: Persistence-Driven Design and Visualization of Morse Vector Fields, at China Vis, July 21 - 24, 2019.

Bei Wang Invited Talk: A Structural Average of Labeled Merge Trees for Uncertainty Visualization, at Dagstuhl Seminar 19212: Topology, Computation and Data Analysis, May 19 - 24, 2019.

Bei Wang Invited Talk: An Introduction to Discrete Stratified Morse Theory, at JMM AMS-AWM Special Session on Women in Applied and Computational Topology, Jan. 26 , 2019.

Bei Wang Invited Talk: Mapper on Graphs and Visual Detection of Structural Changes in Time-Varying Graphs, at VISA Research, Dec. 12 , 2018.

Bei Wang Invited Talk: Topological Perspectives On Stratification Learning, at ICERM TRIPODS Summer Bootcamp: Topology and Machine Learning, Aug. 6-10, 2018.

Year 1 (2017 - 2018)

Bei Wang Conference Talk: Discrete Stratified Morse Theory: A User's Guide, at 34th International Symposium on Computational Geometry (SOCG), June 11-14, 2018.

Bei Wang Invited Talk: Stratification Learning with Computational Topology: Overview, Challenges, and Opportunities, at CG Week 3rd Workshop on Geometry and Machine Learning, June 11, 2018.

Bei Wang Invited Talk: Stratification Learning with Computational Topology, at IMA Workshop Bridging Statistics and Sheaves , May 21 - 25, 2018.

Bei Wang Invited Talk:Topological Data Analysis In a Nutshell, at NII Shonan Meeting Seminar 122 Analysing Large Collections of Time Series, Feburary 12-15, 2018.

Students

Lin Yan (Fall 2017 - Present)
School of Computing and Scientific Computing and Imaging Institute
University of Utah
linyan AT sci.utah.edu

Youjia Zhou (Spring 2019 - Present)
School of Computing and Scientific Computing and Imaging Institute
University of Utah
zhou325 AT sci.utah.edu

Fangfei Lan (Spring 2020 - Present)
School of Computing and Scientific Computing and Imaging Institute
University of Utah
fangfei.lan AT sci.utah.edu

Archit Rathore (Summer 2018 - Present)
School of Computing and Scientific Computing and Imaging Institute
University of Utah
archit.rathore AT utah.edu

Ilkin Safarli (Summer 2020 - Spring 2021, graduated Summer 2021)
School of Computing and Scientific Computing and Imaging Institute
University of Utah

Yaodong Zhao (Fall 2017 - Spring 2019, graduated Spring 2019)
School of Computing and Scientific Computing and Imaging Institute
University of Utah

Acknowledgement

This material is based upon work supported or partially supported by the National Science Foundation under Grant No. 1661375 and 1661348, project titled "ABI Innovation: A Scalable Framework for Visual Exploration and Hypotheses Extraction of Phenomics Data using Topological Analytics."

Any opinions, findings, and conclusions or recommendations expressed in this project are those of author(s) and do not necessarily reflect the views of the National Science Foundation.

Web page last update: October 12, 2021.